Attractor States
A powerful idea that can be found across disciplines and fields.
I was reading The Selfish Gene a couple of weeks ago. Dawkins was talking about evolutionarily stable strategies and the peculiar existence of the prisoner’s dilemma in nature, and that triggered an explosion of connections. Deep Simplicity immediately came to mind. Ideas from physics, chemistry, biology, economics, statistics, flooded in. It was a truly thrilling experience.
In this article, I’ll explore a concept that has transcended all fields and disciplines. I cannot, as of now, do an exhaustive analysis because of how little depth of knowledge I have in these different verticals. However, I believe it vividly captures the power of escaping one’s field and looking for ideas elsewhere. Many of them, with a twist, can be deeply helpful in understanding phenomena beyond what made them rise.
The first time this idea hit me from an unexpected place was in physics, specifically in thermodynamics. The second law of thermodynamics is about the increase of entropy within a closed system. If you leave it isolated, with no interference, the system will tend towards the state of maximum entropy and minimum energy. In a bowl, balls would sink to the middle. This acts as an attractor state.
Evolutionarily Stable Strategies
In the 50s-60s, biologists were studying how animals compete within an ecosystem. When one encounters a problem somewhere, it’s plausible that a similar thing happened elsewhere. If you find out how it’s been dealt with in this elsewhere, you might find some valuable tool for analyzing the situation you are interested in.
Essentially, evolutionarily stable strategies is an idea biologists took from game theory. It’s about thinking of species, or individuals, as players, and trying figure out what’s the soundest strategy each participant should utilize to maximize their chances to reproduce. Let’s imagine a population of one species, where individuals can adopt 1 of 2 strategies:
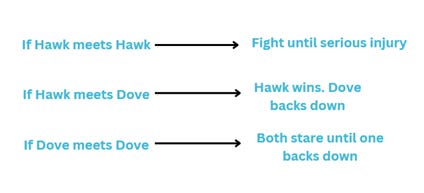
Hawk. Hawks are aggressive. Individuals adopting this strategy will always fight until death or a serious injury when they encounter another individual.
Doves. Doves are easy-going. Individuals adopting this strategy will always do a contest stare with individuals they meet. They never fight.
To make things interesting and exploit the potential for thinking ground this model offers, we assign payoffs to encounters between 2 individuals.
Win: +50
Serious injury: -100
Long stare: -10
The purpose of this exercise is not to infer whether doves or hawks will win. We know for certain that hawks will always win encounters. An evolutionarily stable strategy is the strategy, or set thereof, that, if the majority of the population adopts, there will be no better alternative. It’s impermeable. That’s what we are interested in. Now let’s suppose two scenarios:
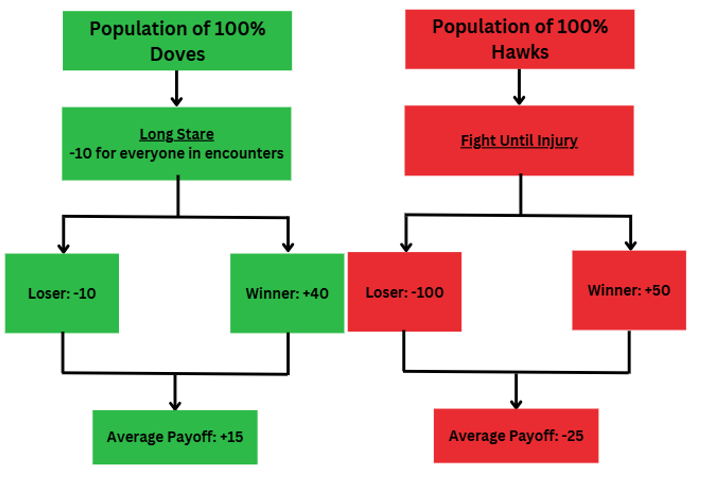
After a quick glance, you would think doves will dominate. But that’s wrong. If a population is full of doves, becoming a hawk turns suddenly profitable. Every time a hawk meets another individual, they’ll win because doves won’t fight. The average payoff for a hawk would be +50, reproducing the most.
Now you think hawks will dominate. But if a population is full of hawks, then becoming a dove turns profitable. Although the average payoff for a dove in a population full of hawks will be -10, hawks’ average payoff will be almost -25. In this scenario, doves will reproduce the most and their share of the population will rise.
It appears as if the ecosystem will follow a pattern of ascension of one strategy to then be succeeded by its decline and full ascension of the other. But there is a fascinating kicker. Mathematically, there is an equilibrium where the population will settle. Every 12 individuals, 7 will be hawks, and 5 will be doves. If a strategy gets used more than that, the other one will become profitable and hence the system will regulate itself. When a population reaches an equilibrium, alternative strategies get penalized.
These “attractor states” is what I’ve seen everywhere. I’ll briefly go over some of these.
In microeconomics, this idea of how players think about their strategies, which are invariably affected by what other players’ options are, reached an interesting conclusion. Where a finite number of strategies can be determined, alongside the payoffs or costs they carry, you can mathematically arrive at the strategy that’s the most convenient for every player, considering other players’. Deviating from it gets penalized and hence you have no incentive to do so.
Where it gets interesting is when you observe systems where individual players choose the best strategy they presumably have, but end up being much worse off than if another set of populational choices were taken. This is classically known as The Prisoner’s Dilemma. Simply stated, when two criminals in this exercise are interrogated, the options they can choose is either to stay silent or defect, betraying the other. Given payoffs and costs, both prisoners’ best strategy is to betray the other, but they’d be much better off if they could cooperate and stay silent.
This is not in and of itself that novel. The exciting part is when Dawkins brings this idea to nature. One would think that in ecosystems where this marvelous agent regulates everything, the attractor state would be the optimal state, yet that’s not what happens. Our hypothetical population would end up settling in 7/12 hawks, yielding an average populational payoff of +6.25. However, if the population was full of doves, the average populational payoff would be much higher, at +15.
In a free market economy, any given good will have a set of suppliers and people who demand it; sellers and buyers. The attractor state is where, on weighted average, these two sets of people agree. Goods will presumably reach an equilibrium, which determines what its supply will be and the price at which it’ll be done so.
The invisible hand is another cornerstone of economics, but most people get this one wrong. Adam Smith spoke about the invisible hand in the context of people doing what’s in their best interests; that by doing so, they end up, more frequently than not, benefiting the whole society. It is as if an invisible hand makes them promote the greater good by promoting their own.
“By directing that industry in such a manner as its produce may be of the greatest value, he intends only his own gain; and he is in this, as in many other cases, led by an invisible hand to promote an end which was no part of his intention. Nor is it always the worse for the society that it was no part of it. By pursuing his own interest, he frequently promotes that of the society”
Staying in economics, equilibriums are everywhere. There’s a peculiar thing called the “law of convergence,” which posits that, as a country gets richer and richer, its per capita gdp growth rate will tend towards 2%. It also has the counterpart that, the lower the base they start with, the higher their potential growth rate, naturally converging to this 2% as they get richer.
This is, in part, just the extrapolation of an incredibly powerful statistics concept. The law of large numbers states that the larger you make a sample of observations/tries over an independent event, the more will the average of the sample tend towards the expected value/true value, if there is one. When flipping a coin, you’ll notice a convergence towards a 50/50 distribution the more coinflips you do.
Regression to the mean, or base rates if I’m not incorrect, derives from the above and probability theory. Given there exists some sort of true value, when considering an event’s outcome distribution, whenever a trial is close to a tail of the distribution, the subsequent trial/observation is more likely to be nearer the true value.
In psychology, I found there are two similar things in this line of attractor states:
We are strongly wired to follow the crowds, which associated term is herding behavior. Hence my vague corollary is that what a society thinks, most individuals will think as they grow up. These generally held beliefs will act as an attractor state of people’s thoughts.
Humans will do what rewarded them in the past, avoid doing what harmed them, and our subconscious continuously speculates about potential payoffs in the systems wherein we operate. Therefore, people’s behavior will be skewed towards where incentives are aligned. That’s why they are so powerful a controller of human cognition. Well defined incentives could be used as accurate predictors of average behavior.
In physics, there’s a thing called centripetal forces. Newton spotted and coined the term in the 1670s when he was investigating gravity. These have some sort of pulling effects. The heaviest object in the solar system, although now I’m getting to dangerous territory, is the sun. That is why planets orbit therearound. It acts as an attractor spot. The system does not tend towards it due to other forces and variables in place but it’s an incredibly peculiar thing to think about.
Then we have thermodynamics, which posits, as aforementioned, that isolated systems will tend towards the state of maximum entropy and minimum energy. If you have two separate boxes, one of which contains atoms of gas, and open the valve, connecting them, atoms will distribute evenly throughout the whole new open space.
Whenever you are making a pile of sand, rice, grain, or stacking one of these little things, you will eventually reach some sort of peak. After such a threshold, each time you add to the pile will cause an avalanche, of varying dimensions. That peak is a critical value.
The last one I will cover in this article is one which we are all familiar with. Ben Graham wrote that, “over the short run, the market is a voting machine but in the long run it is a weighing machine.” The convergence between intrinsic values, or fundamentals, and stock prices follows from this idea that the fundamentals act as an attractor state.
Final Remarks
I believe there are two main takeaways from this array of similarities. Firstly, there are things, system-wise, that can be taken from one discipline to another, with great benefit to those who do. Secondly, it seems impossible to find these things unless you really search. As obvious as it now seems, the multidisciplinarity of attractor states only hit me after reading several books, most of them focused on particular verticals of knowledge.
I hope you all finish the year well and have a great 2025. Thank you immensely for taking the time to read this.
Best wishes, Giuliano